报告题目：Convergence rates in homogenization of parabolic systems with locally periodic coefficients
报 告 人：徐侥（中国科学院数学与系统科学研究院数学所 博士后）
报告时间：2020年7月9日（星期四）下午2点半开始
报告地点：腾讯会议线上报告
会议链接：//meeting.tencent.com/s/RCXnGyfRBFnV
会议 ID：469 636 475
报告摘要：This talk mainly concerns with the quantitative homogenization of second-order parabolic systems with time-dependent locally periodic coefficients in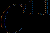 
cylinders, i.e.,
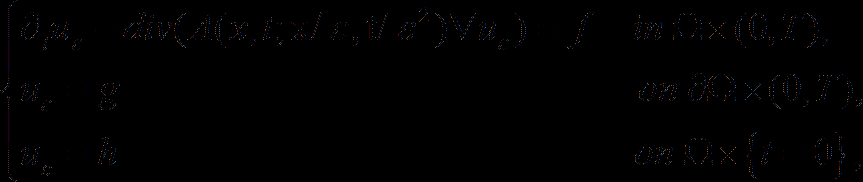
where 
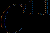is a bounded domain in 
, the matrix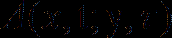 
defined on 
is bounded, elliptic and 1-periodic in 
, and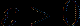 
is a parameter. Under nearly minimum smoothness assumptions on 
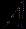which indicate the 
order differentiability in 
and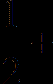 
order differentiability in 
, the sharp-order scale-invariant convergence rate to some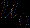 
is established. To do this, we employ fractional derivatives on intervals to build several almost optimal estimates for the macroscopic smoothing operator, and derive a new estimate for the integrals on temporal boundary layers. This extends the previous work [Y. Xu and W. Niu, Comm. Partial Differential Equations, 2020] about elliptic systems with stratified structure.
报告人简介：徐侥，中国科学院数学与系统科学研究院数学研究所博士后。2012年本科毕业于南京大学数学系，并于2019年在南京大学获得理学博士学位，博士期间曾在美国肯塔基大学联合培养两年。现主要从事于偏微分方程均匀化理论的研究工作，系列工作发表在 JFA、CPDE、JDE、AMPA、Asymptot. Anal.、DCDS等国际重要期刊上。
